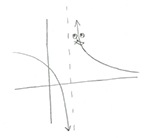
When I was first introduced to asymptotes during my freshman year of high school, I found them hauntingly poetic: a line that a graph will grow closer and closer to but never reach. Rain was battering the windows of the second-floor classroom, and I should have been taking notes, but I imagined a consciousness at the tip of the graph as it shot forever up or down, reaching for something it could never touch.
I’m using the term “poetic” liberally. I’m taking it beyond the mundane, past a set of written lines. Poetry is a kind of abstract beauty that’s difficult to define without going in circles. Part of what gives poetry its beauty is how it evades a definition.
When I looked for it, and sometimes when I didn’t, I found math full of poetry. Parallel lines, forever the same distance apart, never meeting. Graphs of trigonometric functions, cycling over and over. The small pieces of graphs we draw that leave out everything else. We understand where these graphs go – that they stretch on forever, never deviating from the behavior we’ve already figured out – but never bother to follow it further.
We concentrate on the origin, on where functions twist and loop and cross, because the part of a graph where we usually just put an arrow isn’t mathematically relevant. But it’s still there.
What I find so poetic about these is how they all deal, in some way, with infinity. It’s a concept we can understand but can’t truly visualize. Our brains have a beginning and an end – they reach out for a way to capture infinity within that but sometimes, as can happen with math, the limit does not exist. To me, that gap between reality and our ability to visualize it is where poetry lies.

Is poetry, then, our attempt to bridge the gap between what we know and what we can’t understand? The abstract abyss between our minds and something greater? Does that make poets brave explorers of a darkness into which they can bring no light? When Death stopped for Dickinson, did she truly travel toward eternity? Did Whitman’s captain lead him into some plane we do not inhabit in our everyday life?
If I can recognize poetry in asymptotes, does that same beauty exist elsewhere? I started looking. I would smile as I blinked away snowflakes that nestled in my eyelashes like glitter. The hypnosis of routine – showering, driving familiar routes – gave me comfort. I would focus on each contact between my foot and the ground during a run, noticing how the impact spread up my calf like a crack in glass. Without fail, whenever I looked for poetry, I found it.
And within each of the poems was more infinity. The snowflakes were countless. Routines promised to continue indefinitely. Footsteps compounded into miles I never bothered to measure. What made it poetry was the bridge between the tangible and unimaginable. I can control a single snowflake, but a snowstorm is beautiful because I cannot.
I had to limit myself to copying down only the portions of a graph that fit on the screen of my calculator. I yearned to keep drawing, to extend the pencil line to the edge of the page and onto the table. Abbreviating the graphs was an injustice to the true expanse of infinity. But that higher plane of poetry has no place in an algebra II class, and in most of our daily lives for that matter; attempting to reconcile all that existence takes too much effort. Ignoring it, however, does not mean it isn’t there. The poetry is waiting. Creating it requires only recognizing its existence.
I’m not sure that we’re capable of mapping the abyss of poetry. On the graph of our mental capacity, fully visualizing infinity is the asymptote; we can draw closer and closer to it, but never quite reach.